Linear harmonic oscillator derivation

718 where nis the vibrational quantum number and q k. M 1 x 1 ξ 1 m 2 x 2 ξ 2.

42 It comprises one of the most important examples of elementary Quantum Mechanics.

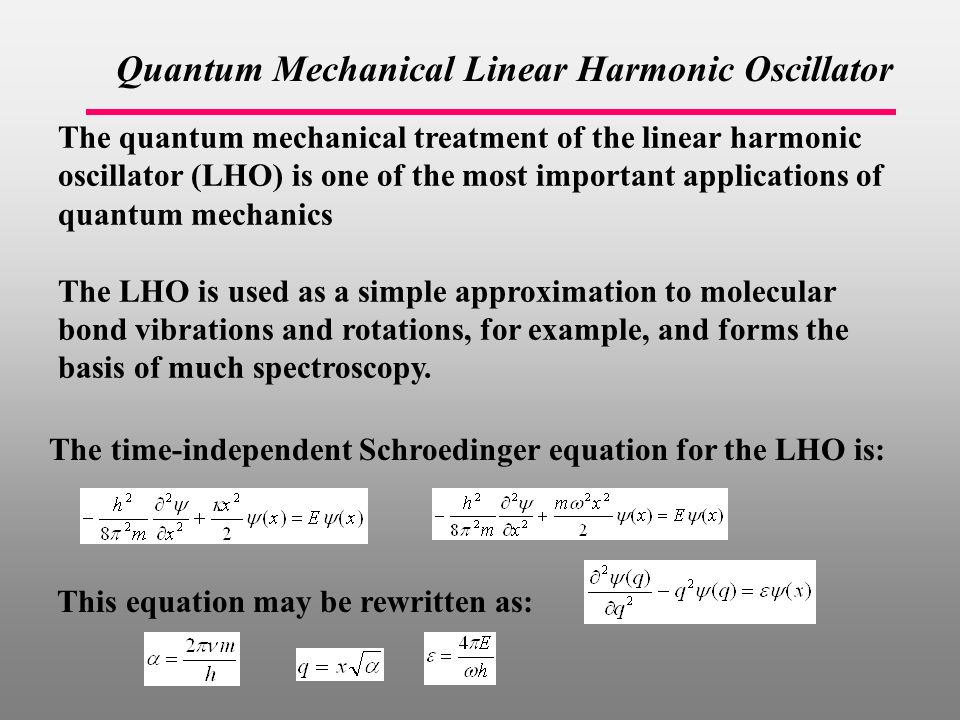
Linear harmonic oscillator derivation. Linear Harmonic Oscillator The linear harmonic oscillator is described by the Schr odinger equation i t xt H xt 41 for the Hamiltonian H 2 2m 2 x2 1 2 m2x2. 42 It comprises one of the most important examples of elementary Quantum Mechanics. There are sev-eral reasons for its pivotal role.

The linear harmonic oscillator describes vibrations in molecules and. Heuristic derivation of the energy levels of a linear harmonic oscillator that involves only algebra. The Harmonic Oscillator as a Particle in a Box We consider a linear harmonic oscillator of reduced mass µ and frequency ω.

The classical Hamiltonian for this oscil-lator is given by p Hxp 2 2µ µω2 2 x2 1. BSc III Paper First Physics. M 1 x 1 ξ 1 m 2 x 2 ξ 2.

Simplifying m 1 ξ 1 m 2 ξ 2. Assume for the linear oscillator the interatomic force is an elastic one. This corresponds to the problem in harmonic approximation.

Then a force acts on any atom that is out of its equilibrium position. F - β ξ 1 ξ 2. Simple Harmonic Oscillator Where.

Cos sin combination of sin and cos. The general solution is a linear 0 0 0 m k y t A t B t ω ω ω. THE HARMONIC OSCILLATOR 31.

Solution by discretization 19 where the n 1-dimensional matrix Cis C µ 1 1 0 0 1 µ 2 1 0. 0 1 µn1 µj 2ǫ2ω2 j. 35 For vanishing ωj the square matrix Cis proportional to the discretized second derivative one-.

Link of linear harmonic oscillator or one dimensional harmonic oscillator in quantum mechanics. Part - 2 video. Damped Simple Harmonic Oscillator If the system is subject to a linear damping force F br or more generally bj rj such as might be supplied by a viscous fluid then Lagranges equations must be modi-fied to include this force which cannot be derived from a potential.

Recall that we had developed the expression d dt µ L q L q. The coherent states of the harmonic oscillator are special nondispersive wave packets with minimum uncertainty σx σp ℏ2 whose observables expectation values evolve like a classical system. They are eigenvectors of the annihilation operator not the Hamiltonian and form an overcomplete basis which consequentially lacks orthogonality.

Linear simple harmonic motion is defined as the motion of a body in which the body performs an oscillatory motion along its path. The force or the acceleration acting on the body is directed towards a fixed point ie. Means position at any instant.

Harmonic oscillator has energy levels given by E n n 1 2h n 1 2. 718 where nis the vibrational quantum number and q k. Note that the energy level are equally spaced and the zero-point energy E 0 1 2.

Wavefunctions of a quantum harmonic oscillator. The energy eigenstates of the harmonic oscillator form a family labeled by n coming from Eφˆ x. Justify the use of a simple harmonic oscillator potential V x kx22 for a particle conflned to any smooth potential well.

Write the timeindependent Schrodinger equation for a system described as a simple harmonic oscillator. Youhavealreadywritten thetimeindependentSchrodinger equation for a SHO in chapter 2. In this work we present a heuristic derivation for the energy levels of a linear harmonic oscillator that involves only algebra.

This derivation offers the instructor a way to rationalize the energy level spectrum of a harmonic oscillator without having to solve the complicated differential equation prescribed by the Schrodinger equation for the harmonic oscillator. The linear harmonic oscillator problem is one of the most fascinating problems in quantum mechanics. It allows us to understand the basic features of a quantum system along with its transition to the classical domain.

It has applications in many problems in physics. LINK OF DEGREES OF FREEDOM VIDEOhttpsyoutubekC_5khgd9kELINK OF CONSTRAINTS AND CONSTRAIND MOTION. Hello friends here i have discussed simple harmonic oscillator its differential equations and solution.

Hope u got this wellFor more videos o. LINEAR SIMPLE HARMONIC OSCILLATOR LHO Horizontal oscillations of a spring-mass system. Consider a system containing a block of mass m attached to a massless spring with stiffness constant or force constant or spring constant k placed on a smooth horizontal surface frictionless surface as shown in Figure 1013.

Let x 0 be the equilibrium position or mean position of mass m when it is left.