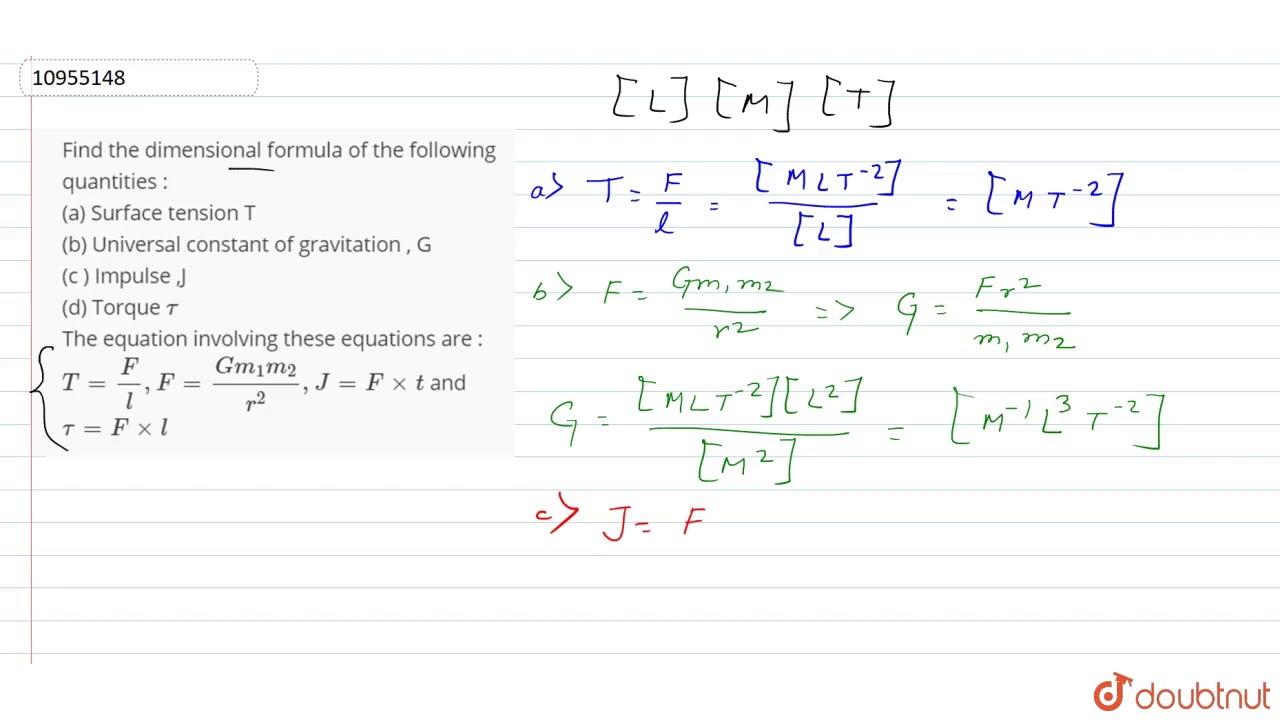
Dimensional Formula of Surface Tension. Such rods elongate under tension.

A tension is a force along the length of a medium especially a force carried by a flexible medium such as a rope or cable.
Dimension of tension in physics. The Tension in Three Dimensions. Tension is also used to describe the force applied by the ends of a three-dimensional continuous material like the truss and rods. Such rods elongate under tension.
The amount of lengthening and the load will cause failure and both will depend on the force per cross-sectional area rather than the force alone so stressaxial forcecross-sectional area. The Tension in Three Dimensions. Tension is likewise used to portray the force applied by the end of a three-dimensional consistent material for example a pole or support part.
Such a bar lengthens under tension. The measure of extension and the heap that will cause disappointment both to rely upon the force per cross-sectional territory as opposed to the force alone so stress pivotal forcecross. In physics tension is the force exerted by a rope string cable or similar object on one or more objects.
Anything pulled hung supported or swung from a rope string cable etc. Dimensional Formula of Surface Tension. The dimensional formula of surface tension is given by M 1 L 0 T-2.
Where M Mass. Surface Tension T Force Length-1. 1 Since Force Mass Acceleration.
And acceleration velocity time-1 L T-2 The dimensional formula of force M 1 L 1 T-2. A tension is a force along the length of a medium especially a force carried by a flexible medium such as a rope or cable. The word tension comes from a Latin word meaning to stretch.
Not coincidentally the flexible cords that carry muscle forces to other parts of the body are called tendons. 107 Zeilen The dimensional formula is a compound expression showing how and. DIMENSIONS Dimensions of a physical quantity are the powers to which the fundamental quantities must be raised to represent the given physical quantity.
Force Quantity mass acceleration mass velocity time mass length time 2 mass length time2 So dimensions of force. 1 in mass 1 in length 2 in time. We can derive dimensional formulas of physical quantities from their units alsoHence the unit of planks constant is Joule-sec JouleUnit of Energy DF is ML2T-2 and secUnit of time DF is T.
If we multiply these two ML2T-2T ML2T-1Similarly Coefficient of viscosity. DF of Stress is M L -1 T-2 and DF of Velocity is reciprocal of time ie T-1Therefore DF Of Coefficient of. Rules for writing dimensions of a physical quantity.
Force F MLT-2 Velocity. V LT-1 Charge. The dimension of a physical quantity is defined as the power to which the fundamental quantities are raised to express the physical quantity.
The dimension of mass length and time are represented as M L and T respectively. We say that dimension of velocity are zero in mass 1 in length and -1 in time. Dimensional formula of a Physical Quantity.
The dimensional formula is defined as the expression of the physical quantity in terms of its basic unit with proper dimensions. Tension is a force working along the length of a medium especially this force is carried by a flexible medium like a rope or cable. The flexible cords which carry muscle forces to other parts of the body are known as tendons.
Any flexible connector like a string rope chain wire or cable can exert pulls only parallel to its length. Dimension of Surface Tension. As we know surface tension is given by the formula Surface tension FL.
We know that F ma substituting the value in the equation we get maL. Equating the fundamental quantities into the equation we get MLT-2 L-1. Solving further we get MT-2.
Hence the dimensional formula of surface tension is MT-2. The dimension of a physical quantity are the powers to which the fundamental or base quantities like mass length and time etc. Have to be raised to represent the quantity.
Consider the physical quantity Force. The unit of force is Newton. 1 Newton 1 kg msec2.
The dimension of a derived unit like velocity which is distance length divided by time then becomes hboxLT-1 in this notation. The dimension of force another derived unit is the same as the dimension of mass times acceleration and hence the dimension of force is hboxMLT-2. Let us find the dimensions of the terms in 1.